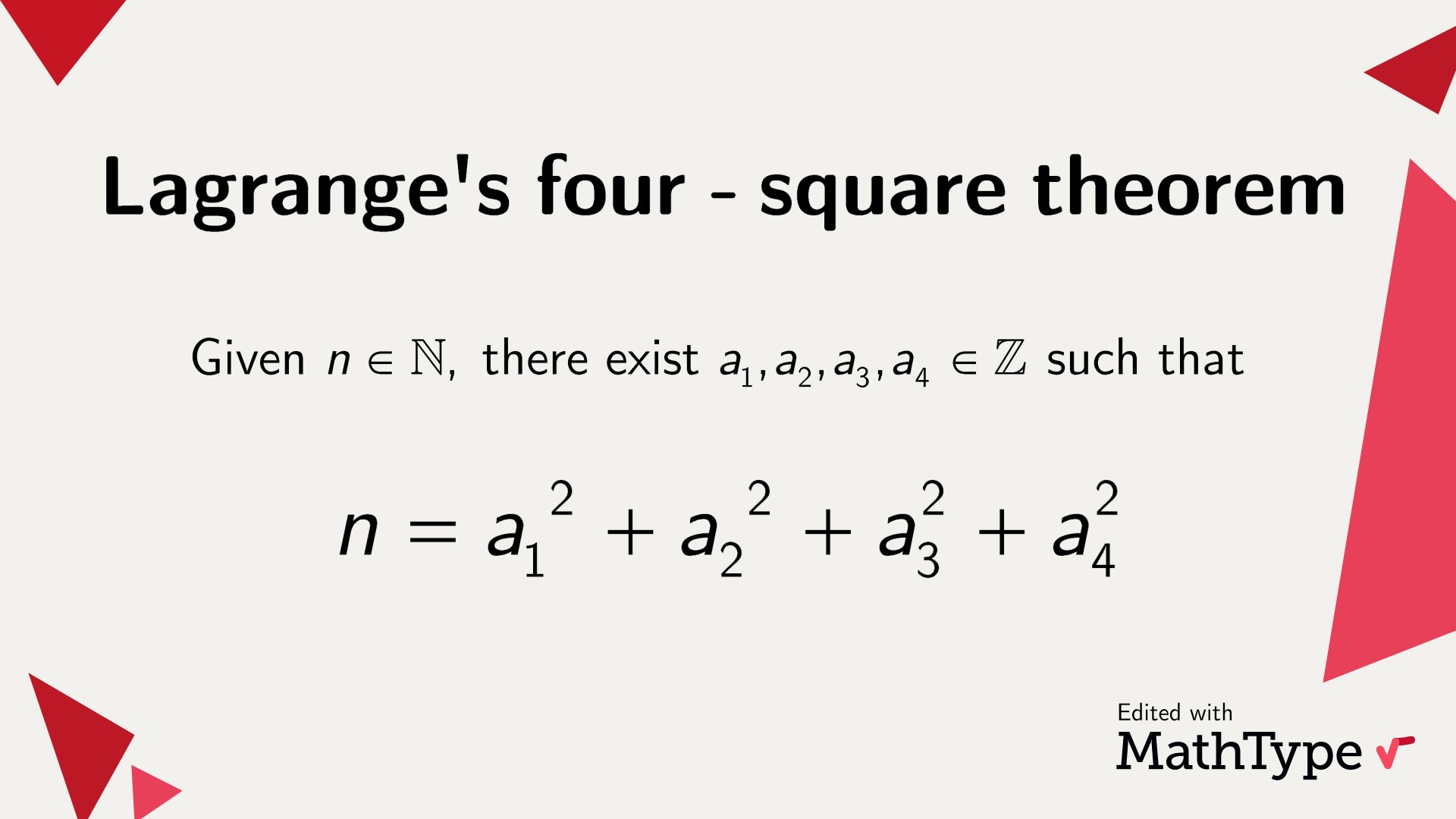Casa
four square theorem

PDF) Quarternions and the Four Square Theorem

Por um escritor misterioso

Descrição
The Four Square Theorem was proved by Lagrange in 1770: ev- ery positive integer is the sum of at most four squares of positive integers, i.e. n = A2 +B2 +C2 +D2;A;B;C;D 2 Z An interesting proof is presented here based on Hurwitz integers, a subset
PDF) Quarternions and the Four Square Theorem
General Index Theory: Its Mathematical and Physical Structures - GRIN
PDF) Quarternions and the Four Square Theorem
Lagrange's four-square theorem - Wikipedia
PDF) Quarternions and the Four Square Theorem
Sums of four squares — AnhThu Nguyen
PDF) Quarternions and the Four Square Theorem
Minimal non-abelian nodal braiding in ideal metamaterials
PDF) Quarternions and the Four Square Theorem
Quaternions in Space
PDF) Quarternions and the Four Square Theorem
Integrated Sensing and Communications With Reconfigurable Intelligent Surfaces
PDF) Quarternions and the Four Square Theorem
Online deep Bingham network for probabilistic orientation estimation - Li - IET Computer Vision - Wiley Online Library
PDF) Quarternions and the Four Square Theorem
1.28: Sum of Squares - Mathematics LibreTexts
PDF) Quarternions and the Four Square Theorem
Page:Scientific Papers of Josiah Willard Gibbs - Volume 2.djvu/130 - Wikisource, the free online library
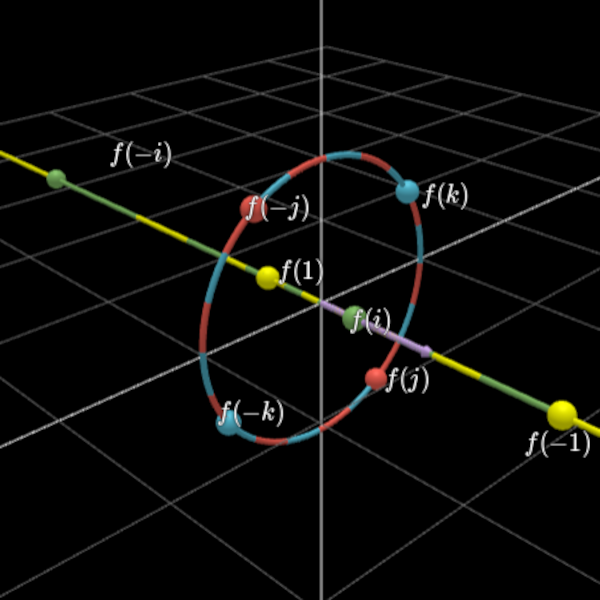
PDF) Quarternions and the Four Square Theorem
Today's The Day You Finally Learn Quaternions
PDF) Quarternions and the Four Square Theorem
Full article: Efficient data encryption technique using quaternions for wireless sensor network
PDF) Quarternions and the Four Square Theorem
Lagrange's four-square theorem - Wikipedia
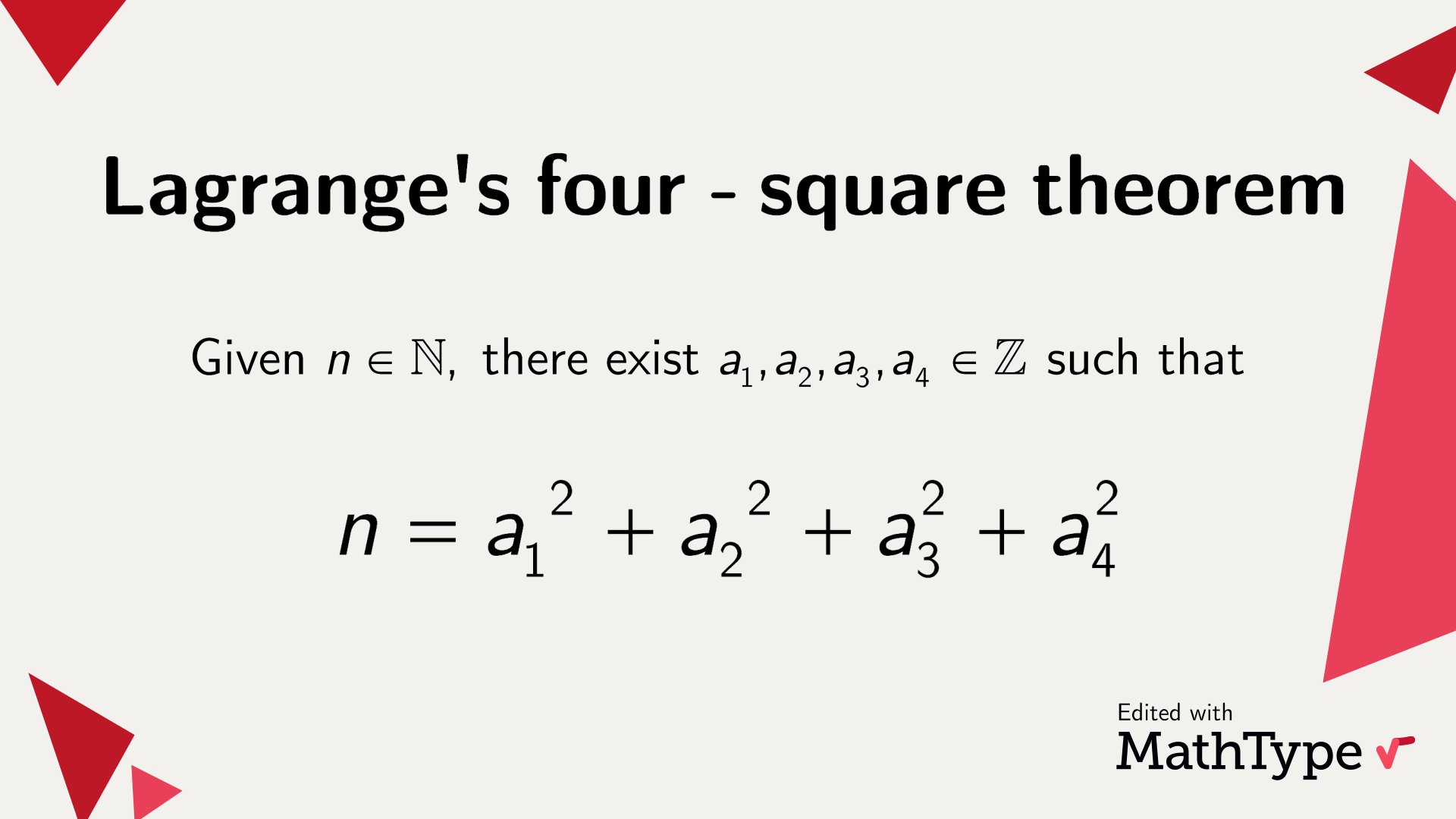
PDF) Quarternions and the Four Square Theorem
MathType on X: Lagrange's four-square theorem asserts that any positive whole number can be written as the sum of four squares of integers. Leave an example in the comments! #MathType #NumberTheory #math #
PDF) Quarternions and the Four Square Theorem
PDF] Circular and hyperbolic quaternions, octonions, and sedenions—Further results by Kevin Carmody · 10.1016/s0096-3003(96)00051-3 ·
PDF) Quarternions and the Four Square Theorem
On the Continuity of the Generalized Inverse
de por adulto (o preço varia de acordo com o tamanho do grupo)